A 20 through 10 meter 43-foot Vertical Doublet

I'm not a contester, but a good contest always provides an opportunity for working DX, even for those of us who aren't running kilowatts and stacked yagis.

Next weekend (March 7th, 2015) is the ARRL's DX Contest (Phone).  I'll be up at the "portable 6" location during that weekend, and I would normally use my full-wave 80 meter loop that I have up there.  But the loop, being an oddball shape due to support-tree locations, not too surprisingly has oddball patterns.  For example, here's its azimuth plot on 10 meters:

Some nice gain in some directions.  In others...not so much.  Although it hasn't been a big issue working DX during contests, I have noticed the nulls in the pattern.  So I thought...why not try something else, for comparison?

Given the short time frame between antenna installation and actual contest, my design constraints were:

1. Easy to install.
2. 20, 15, and 10 meter operation (and preferably the WARC bands, too).
3. There's no tower on site, so pattern should be omnidirectional (to fill in the existing loop's holes).
4. Some gain, whenever possible (maximize gain on 10 meters, if possible).
5. Temporary installation, so must disassemble easily.

Horizontal antennas (e.g. dipoles, yagi's, etc.) were pretty much precluded due to their directionality -- I have no way to turn them.  And installing multiple dipoles seemed problematic due to support-tree locations.

So I started looking at verticals.

Ground mounted verticals all seemed to have either fairly high-angled radiation patterns or low gain.  But because there are some very high trees at the QTH here (100 feet, plus), why not raise a vertical up and get it as far off the ground as I could?  Of course, it would then no longer be a quarter-wave vertical, but a dipole of some sort.

I also wanted to try to maximize the gain on 10 and 15 meters with low angles of radiation.  So I started playing around with vertically mounted dipoles (i.e. doublets), varying their heights and lengths using EZNEC, the antenna simulation program.

43 feet (21.5 feet on each side of the feedpoint) seemed like a great length.  10 meter gain peaked at about 6.9 dBi at 7 degrees elevation (a very nice low angle!), compared to other doublet lengths I tested (which were from 28 to 53 feet, in 5 foot increments).

Here are the EZNEC windows...

(click on image to enlarge)

Note that the source is placed in the center of the antenna.  When I later use EZNEC to calculate SWR, this will be the point at which it calculates SWR:  at the antenna itself.

 (click on image to enlarge)

 (click on image to enlarge)

 ...and EZNEC plots (click on images to enlarge):

 

6.9 dBi at 7 degrees.  Not bad!

Being 43 feet long, the antenna itself was non-resonant on all of the ham frequencies, so it would have a high SWR. Clearly, it should be feed with ladder-line (rather than coax) to minimize SWR-related power loss.  But how long should the line be?

There's actually a science to determining feed line length (although sometimes you have to go with what you have on hand, as I did with the feedline to my 80 meter loop).  Depending upon the antenna, certain feedline lengths will produce low SWRs on multiple ham bands.  The G5RV (designed by Louis Varney in 1946) is probably the best known example of this type of multiband antenna.  The ZS6BKW doublet is another example, as is W5DXP's no-tuner antenna.

So what's the science?

The goal is to get the SWR in the ham bands (as seen by the antenna turner) down to a low enough level to allow the tuner to get it the rest of the way down to 1:1 (hopefully without too much power lost in the tuner).

Because the antenna itself is non-resonant on the ham bands, its impedance (and thus SWR) at ham-radio frequencies will be high.  Coax can have significant loss at higher frequencies (when operating with a high SWR), plus it's fairly heavy, which makes it a poor choice to feed my vertical-doublet suspended between trees.  Ladder-line has lower loss and is lighter weight -- it's a much better choice, and the fact that its characteristic impedance is much higher than 50 ohms works in our advantage, too.

Let's see how.  If I were feeding the doublet with 50 ohm coax, EZNEC calculates that the SWR at 28.5 MHz would be 80.8:1 (!!!).  With 400 ohm ladder line this becomes a more reasonable (but still high) 12.3:1.

So we are already ahead, compared to 50 ohms, but 12.3:1 is still pretty high.  I'd like my tuner to see something lower.  And I can do this by intelligently selecting the length of 400 ohm ladder line.  I'll use the Smith chart below to demonstrate how:

(click on image to enlarge)

First, a few points about Smith Charts...

It's important to understand that the Smith Chart can be thought of as an overlay over a plot of the Reflection Coefficient (referenced to a Zo of the user's preference).  The reflection coefficient (also known as Γ (the Greek Gamma)) can be expressed either as a complex quantity using Cartesian coordinates (x-axis = real component, y-axis = imaginary (or reactive) component) or as a polar coordinate.  In the image above the point I've selected is at 0.5355 - j0.6, or, expressed in polar coordinates, 0.85 magnitude, -51 degrees; both are equivalent.

In the Smith Chart above, the outer circle designates a reflection coefficient of magnitude 1 (infinite SWR).  At the "0 degree" point on this outer circle (x-axis, right-hand side), the coordinates can be expressed either 1 + j0 (x,y axis) or as 1, 0° (polar), both are equivalent.  This point corresponds to a load of infinite resistance and no reactance.  That is, an unconnected transmission line.

At 180 degrees the coordinates are -1 + j0 (x,y axis) or 1, 180° (polar).  This point represents a load of 0 ohms resistive and no reactance.   In other words, a short at the end of the transmission line.

At 90 and -90 degrees on the outer circle the coordinated can be written either as 0+j (or 0, 90 degrees), or 0-j (or 0, -90 degree), respectively.  So the values along the y-axis are solely reactive with no resistance.

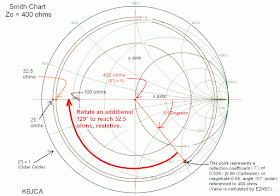
The center of the Smith Chart is our reference Characteristic Impedance (Zo) used to calculate the reflection coefficient, Γ.  In this example, Zo is the impedance of the ladder line I'm using (400 ohms:  JSC 1315).  And at this center point the reflection coefficient is 0; that is, a perfect match to 400 ohms.

I've also added two interesting SWR circles to the chart.  One is for an SWR of 4:1.  It intersects the left-hand x axis at 100+j0 (i.e. 100 ohms, resistive).

The next is an SWR circle of 16:1 intersects the circle at 25 ohms, resistive.

I chose these circles for a specific purpose.  First, the doublet's SWRs that EZNEC calculates at 14.2, 18.1, 21.3, 24.96, and 28.5 MHz are bounded between the inner 4:1 and outer 16:1 SWR circles.

And second (and perhaps the most important), the impedance values where these circles intersect the left-hand x axis lie between 25 and 100 ohms, resistive  -- that is, these SWR circles, if the proper lengths of transmission line are chosen, will result in an SWR of lower than 2:1 (referenced to 50 ohms) at the "transmitter" end to the transmission line.  And it is to this end of the ladder line, now presenting a low SWR relative to 50 ohms, that I can connect my 50 ohm tuner, SWR meter, and transmitter.

So how long does the ladder line need to be to transform the antenna's impedance to the lower, friendlier impedance on the left-hand x axis?

It's actually easy to calculate.  First, recognize that EZNEC, when it calculates SWR, returns the reflection coefficient along with the SWR.  For example, for a Zo of 400 ohms at 28.5 MHz, EZNEC expresses the reflection coefficient of my vertical doublet  in both Cartesian and polar coordinates: 0.5355-j0.66 and magnitude 0.85, angle of -51°, respectively.

(click on image to enlarge)

You can see this point on the Smith Chart below.  I've also shown its SWR circle (12.3:1).  Note that it intersects the left-hand x axis at 32.5 ohms, which would result in an SWR of 1.5:1 in a 50 ohms system.

(click on image to enlarge)

Okay, a quick Smith Chart digression:  if a transmission line is lossless (they aren't but for the moment we will assume they are), as we increase the length of the transmission line, we'll move clockwise around a Smith Chart's circle of constant SWR.  For example, if the transmission line is lengthened by (λ/4)*Vf (where λ is wavelength and Vf is the transmission line's Velocity Factor), we will rotate 180 degrees around the SWR circle.  And if the transmission line is lengthened by (λ/2)*Vf (that is, a half-wavelength), we will rotate through a full 360 degrees and wind up where we started.

Now back to the problem.  The angle of the reflection coefficient, at the antenna, is -51 degrees, and it lies on the "SWR = 12.3" circle. To get to 32.5 ohms, resistive, the ladder line needs to be long enough to rotate the angle of the reflection coefficient by an additional 129 degrees clockwise (actually, 129.05 degrees).  So how long should the line be to achieve this rotation?

Knowing that 180 degrees of rotation equals a change of length of (λ/4)*Vf (see the earlier paragraph), I can set up a simple ratio, where x is the length I'm solving for:

Angle-to-rotate/180 = x / ((λ / 4) * Vf)

Rearranging to solve for x:

x = (Angle-to-rotate / 180) * ((λ / 4) * Vf)

We know that the "angle-to-rotate" is 129 degrees.  The solution is straight forward, but for one small snag specific to my JSC ladder line:  The JSC website does not specify the Velocity Factor of its 1315 ladder line.  Well, all is not lost; I can take a swag at it.  If I look at Wireman 522 ladder line (also with 16 AWG stranded wire), its Vf is 0.91.  Googling shows other ladder line with a Vf of  0.85.  Let me take the average of these two, 0.88, as the Vf.  Using this value, the result for x is:

x = 5.45 feet

So if I feed the antenna with 5.45 feet of 400 ohm ladder line, I should see 32.5 ohms at the ladder line's other end (when measured at 28.5 MHz) if, as I hope, the Vf is 0.88..  If I then add ladder line in lengths that are a multiple of (λ/2)*Vf (in this example, 15.19 feet), the resulting impedance that I measure at the end of the transmission line will still be 32.5 ohms.

I've set up an Excel spreadsheet to easily calculate the lengths of transmission line that intersect the left-hand x axis of the Smith Chart.  You'll see two different sets of calculations below.  One is for a Vf of 0.85, the other is for a Vf of 0.88.

(click on image to enlarge)

Notice the groupings in the cells with common colors.  If the ladder line length is about 51 feet (for a Vf of 0.88), we'll intersect the Smith Chart's left axis (or be close to it) for 20, 15, and 10 meters.  Perfect for my contest application!

Except...51 feet is too short to reach the house.  Nix that.

The next grouping is at about 83 feet of line.  Looks pretty good on 20, 17, and 10 meters, but not so much on 15.  (I actually tried this length.  If I compared the 80 meter full-wave loop to the vertical doublet, background noise was roughly equivalent between the two antennas on all bands except on 15 meters, where background noise was way down on the vertical doublet (ditto with signals), indicating to me it was performing poorly.)

Well, for the contest I really want to have 15 meters, too.  So nix 83 feet.

So on to about 112 feet (there's probably plus/minus a foot or two of measurement error on my part).  Hook it up -- sounds pretty good!  15 meters is alive again.  And Japan coming in stronger on the doublet than on the 80 meter full-wave loop.  A good sign!

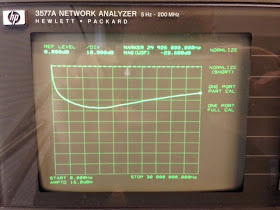
Let's measure SWR Minima, scanning from 13 MHz to 30 MHz with an MFJ-259B SWR Analyzer:

(click on image to enlarge)

SWRs look good!  A little bit high on 17 meters, but that's to be expected if we look at the values in the earlier table.  Its preferred transmission-line length is an outlier compared to the other frequencies, and wants to be shorter.

Notes on installation:

Raising this antenna was a one-man effort.  All in all, it went well.  Not difficult, but definitely time consuming.  Here's the antenna, finally up:

(click on image to enlarge)

(Actually, it's about 43 feet from the lower insulator to ground, not 45).

All support lines use 3/16 inch dacron polyester rope, launched into trees using my pneumatic antenna launcher.

Reeling in 3/16 inch dacron polyester rope up and over a tree using the 30 lb test line on my launcher really felt like I was pushing it, so I decided to go to an intermediary step and use some flourescent-yellow string I'd purchased at some time in the distant past from the hardware store.  So now I'd shoot my 30 lb test line over the tree, then attaching the string to its far end and reel it in.  When I'd gotten the string back to the reel I'd then tie the 3/16" Dacron rope to its end and pull the rope up and over.

At the far end, I made a simple jig for unspooling using about a three foot length of threaded rod to hold spools of rope (or the string).

I discovered that reeling in the string by hand was much too slow, so I made a quick fixture with a drill to speed things up:

I hung the ladder line in loose coils on a rope under the eaves to keep it off the ground.  (If the trees had been farther from the house, I probably could have skipped this step.)

I installed a Common-Mode choke (a.k.a current-mode balun) at the point where my 16 feet of 50 ohm coax emerges from the shack and connects to the 400 ohm ladder line.

I made the CM choke by wrapping 11 turns of RG-142 around two FT240-61 cores, with the short end of its coax connected to the ladder line.

I did a quick check of its impedance by measuring S21 from BNC-shield at one end of the coax to the BNC-shield at the other end (because this inductance is, essentially, the "leakage path" around the CM choke (a good reference:  W7EL balun)).  Here's a photo of the test setup:

After first normalizing S21 by connecting to a long wire between the two red clips above and, well, normalizing the reading, I measured S21 of the CM choke:

Horizontal divisions are every 3 MHz from 0 MHz.  Vertical divisions are 10 dB per.  So that's about 24 dB of loss at 30 MHz, referenced to 50 ohms.

(Note -- I could have used S11, too, to measure impedance of the coiled cable.)

How effective will this choke actually be?  Well, it depends upon the common-mode impedance that it sees at its terminals, and I don't know what this is.  One quick test would be to measure the currents on the ladder line just after the choke.  If the choke is doing its job, these currents should be equal.

In other words -- a test for the future, after I build a current probe!

(And I'll add, regarding where the choke should be in the line -- it should be placed where the common-mode impedance that it sees is low, because it won't attenuate the common-mode signal much if the common-mode load it sees is high.  If I assume that the common-mode impedance, at the antenna itself (relative to ground far below it) is high, then this implies to me that the choke should be placed on an odd-multiple of  λ/4 back from the antenna (i.e. a low-impedance common-mode point).  One potential issue in determining this position, though:  because the signal is common-mode, I doubt the common-mode velocity factor on the transmission line is the same as the differential velocity factor (which is 0.88, or so).  I don't know what it is, and  I'd be interested to hear others' thoughts on this topic).


Final Thoughts...

This design shows that it's possible to design an antenna with a fixed feedline length that will perform on multiple ham bands without a tuner.  But in this example the feedline needs to be about 112 feet in length.

This length, if we assume feedline characteristics similar to Wireman 552 ladder line (it also has 16 AWG stranded wire, like the JSC ladder line I'm using) has a loss of about 1.5 dB on 10 meters.  Not a lot, but...could we make this less?

Also, 112 feet is a lot of ladder line, much more than I actually need in my application to get from antenna to shack (as you could see in the photo above, with the ladder line coiled in loops along the side of the house).  Definitely a negative on the wife/girlfriend acceptance factor!

And one more thing, SWR isn't great on 17 meters.  So, although close, we haven't exactly hit the target.

If I were to make this antenna permanent, I would actually change how I feed and tune the doublet.  I'd still use ladder line to the antenna itself (in fact, I'd be tempted to make open-wire ladder line to reduce loss/detuning when the line is wet), but I'd keep the run of ladder line short -- just long enough to reach my short length of 50 ohm coax that runs through the house wall from the shack to the outside.

At this junction between coax and ladder line I'd add way to remotely (from the shack) insert, in series between the ladder line and the coax, 1, 2, 4, and/or 8 foot additional lengths of ladder line.  This would let me selectively add a delta to the length of the ladder line from 0 feet to 15 feet, in 1 foot increments, allowing me to tune the length of the feedline for minimum SWR (note that 15 feet is very close to a quarter-wavelength on 20 meters, assuming a Vf of about 0.88)


Resources:

EZNEC

K6JCA Antenna Launcher

JSC Ladder Line

Wireman Ladder Line

Wireman Transmission Line Loss Calculator

W7EL Balun Discussion

Smith V3.10 Smith Chart Program


Standard Caveat:

I easily could have made a mistake in the above posting.  If anything looks wrong or is unclear, please let me know.

Also -- the antenna and transmission line were hastily designed  -- there might be lengths of antenna and/or line that are better suited -- I did not do an exhaustive analysis.  Let me know if you have thoughts on this.

Also, use common sense when installing antennas.  Beware of power lines, and, when shooting lines into trees, keep in mind that free-falling objects could drop on your head (or car!) from above.