RF Directional Bridge: Operation versus Source and Detector Impedances

During recent correspondence with Owen Duffy, VK1OD, we discussed RF Directional Bridges and their design.

One question that arose was the effect of the values of the various bridge resistances on a bridge's operation, in this instance the effect of bridge Source and Detector impedances on directional bridge measurements.  

In the various bridge circuits I've seen, Source and Detector impedances (Rs and Rd) seem to ubiquitously be set to Zo (the bridge's reference impedance, typically 50 ohms).  But why?  I could see why this would be the case if one were using an external signal generator with a defined source impedance of 50 ohms as the signal source and measuring the resulting bridge voltage with an external detector having an input impedance of 50 ohms.

But suppose I were designing my own circuit incorporating source and detector with the bridge.  Was it a design requirement that Rs  = Rd = Zo?

As it turns out, no, this is not a design requirement.

Rs and Rd need not equal Zo, the bridge's reference impedance, but their values must satisfy the following equation, assuming all other bridge resistor values equal Zo:

Rs*Rd = Zo^2

This blog post will explain why.

RF Directional Bridge Circuit:

The classic RF Directional Bridge circuit, also known as a Return-Loss-Bridge (or RLB), is shown below:

This circuit is basically a Wheatstone Bridge.  As drawn, above, ZL is the unknown load impedance to be measured, located in the upper right-hand arm of the bridge.  (And although GND is shown at the bottom node of the circuit, it need not be assigned to that node, or to any node at all.)

The bridge is driven by a voltage source Vs with a source impedance Rs (I used Rs in lieu of Zs because it really is a resistive-only impedance).  The source impedance has been set to equal Zo, the bridge's Reference impedance.

The resistors in the bridge arms, R1, R2, and R3, all equal Zo, the bridge's Reference impedance.

Voltage measurement is made across the Detector's impedance, Rd (also equal to Zo).  This measurement is voltage 'Vdet', and its value is proportional to the Reflection Coefficient of ZL, referenced to Zo.

That is,

Vdet = A*(ZL - Zo)/(ZL + Zo)

where 'A' is a constant, and the quantity (ZL - Zo)/(ZL + Zo) represents ZL's Reflection Coefficient, also known as Gamma (Γ).

Directional Bridge Design Requirements:

There are two design requirements for Directional Bridges (that I am aware of):

1.  The "source impedance" looking back into the port to which ZL would be attached (but not currently attached-to) should equal Zo.

2.  The Vdet voltage if ZL = Zo should be 0.  And Vdet should be equal and opposite for ZL values of 0 and Infinity.

The bridge above satisfies these two requirements.  If we remove ZL and calculate (or simulate) the impedance looking into the port to which it had been attached, it equals Zo.  And, given Vs = 1 volt, Vdet is 0v for ZL = Zo, -0.5v for ZL = 0 ohms, and +0.5v for ZL = Open (infinite ohms).

An Equation for Vdet:

To understand how a Directional Bridge's component values affect its operation, we should derive an equation for Vdet that is a function of these components.  We can do this using basic circuit analysis of the circuit, shown below, which results in four equations (also shown below) that we can solve for Vdet:

Pushing pencil on paper to derive an equation for Vdet from these four equations, for me, quickly became prone to error, and so I turned to MATLAB's Symbolic Math Toolbox to ease the pain with the following MATLAB script:

The equation it returned was a complicated one:

vdet = -(Rd*Vs*(R1*R3 - R2*ZL))/(R1*R2*R3 + R1*R3*Rd + R2*R3*Rd + R1*R2*Rs + R1*R3*Rs + R1*Rd*Rs + R2*Rd*Rs + R3*Rd*Rs + R1*R2*ZL + R1*R3*ZL + R2*R3*ZL + R1*Rd*ZL + R2*Rd*ZL + R2*Rs*ZL + R3*Rs*ZL + Rd*Rs*ZL)

Slightly rearranging the equation:

Vdet = (Rd*Vs*(R2*ZL - R1*R3)) /

        (ZL*R1*R2 + ZL*R1*R3 + ZL*R2*R3* + ZL*Rd*Rs +

         ZL*R1*Rd + ZL*R2*Rd + ZL*R2*Rs + ZL*R3*Rs +

         R1*R3*Rd + R2*R3*Rd + R1*R2*Rs + R1*R3*Rs +

         R1*Rd*Rs + R2*Rd*Rs + R3*Rd*Rs + R1*R2*R3)

Our goal is to take the above equation and from it produce a final equation in the form of:

Vdet = A*(ZL - Zo)/(ZL + Zo)

where 'A' is a constant, and the quantity (ZL - Zo)/(ZL + Zo) represents ZL's "Reflection Coefficient", also known as Gamma (Γ).

The numerator Rd*Vs*(R2*ZL - R1*R3) is already in the form of a Constant times (ZL - Zo), if we assume R1*R3 = R2*Zo, in which case the numerator becomes:  Vs*Rd*R2*(ZL - Zo).

Note that if R1 = R2 = R3 = Zo, then the requirement that R1*R3 = R2*Zo is satisfied.

So all we need to do is to whip the denominator into the shape of a second Constant times (ZL + Zo).  You can see that the 16 denominator terms have been grouped into 8 that contain ZL and 8 that don't contain ZL -- the latter will become, in some way, the 'Zo' part of the equation (ZL + Zo).

Arriving at Vdet's Relationship with Rs and Rd, the Source and Detector Impedances:

From LTSpice simulations it appeared that the values of Rs and Rd resulted in a circuit that met the two "Directional Bridge Design Requirements" mentioned, above, if the values of Rs and Rd satisfied the following equation:

Rs*Rd = Zo^2

and with R1 = R2 = R3 = Zo.

But a demonstration with LTSpice is not a mathematical proof.  This goal is easily achieved, though, by substituting Rs*Rd = Zo^2 and R1 = R2 = R3 = Zo into the above equation for Vdet.  Doing so, the Vdet equation reduces to:

Vdet = (Vs*Rd/(2*(2*Zo + Rs + Rd)))*((ZL - Zo)/(ZL + Zo))

We can see that this equation is now in the form of 

Vdet = A*(ZL - Zo)/(ZL + Zo) = A*Γ

where A = Vs*Rd/(2*(2*Zo + Rs + Rd)).

An LTSpice Simulation Example:

Let's let Zo = 50 ohms, Rs = 1 ohm and Rd = 2500 ohms (thus satisfying Rs*Rd = Zo^2), and Vs = 1 volt.  Here are the results of an LTSpice simulation of this circuit:

You can see that the port impedance measured looking into the port to which ZL would attach is 50 ohms, and that Vdet = 0 when ZL = Zo, and +/- 0.481 volts when ZL = Infinite or 0 ohms, respectively.

Taking it to the Limit:

So now we know that Rs and Rd need not always equal Zo.  What happens if we take these two values to their limits by reducing Rs to 0 and increasing Rd to infinity (i.e. we remove Rd)?

The equation for Vdet reduces to:

Vdet = (Vs/2)*(ZL - Zo)/(ZL + Zo)

We will see something interesting if I add a signal-port (and ground) in lieu of ZL and rearrange this circuit by flipping all components (except for the voltage source) upside down to become:

I've replaced ZL with a test port to which a load (ZL) would attach.  If a transmission line were attached to this port, the voltage at the R3/Port node would represent the sum of the forward voltage wave's amplitude (Vf) and the reflected voltage wave's amplitude (Vr) at this point.

That is, the voltage at the test port equals Vf + Vr.

Note that Vf travels out the ZL port onto the transmission line, and Vr, representing reflection of Vf from the far-end of the transmission line, comes into the port from the outside world.

Vr, arriving at the port from the other end of the transmission line, sees R3 as the transmission line's near-end termination.  And so its power absorbed, with no re-reflections, by R3, because the latter's value equals the transmission line's Zo.

The voltage at the R1/R2 node is simply a simulcra of Vf traveling out the ZL port.  Assuming the ZL port is attached to a transmission line of Zo and that there are no re-reflections of Vr from R3 (because R3 = Zo), Vf will always be Vs*R3/(Zo+R3), or Vs/2, given R3 = Zo, irrespective of the value of Vr.

Given that R1 and R2 both equal Zo, the voltage at their common node is also Vs/2, the same as Vf going out the port.  

Vdet is the difference between these two nodes, or 'Vf+Vr' - Vf.  The result is:  Vdet = Vr.

One of the definitions of Reflection Coefficient is  Γ = Vr/Vf.  So if we normalize our measured Vr by Vf (the voltage at the R1/R2 node), we will have measured Gamma, the Reflection Coefficient of the impedance ZL, measured at the test port.

We arrive at the same result if we used our previous equation, derived above:

Vdet = (Vs/2)*(ZL - Zo)/(ZL + Zo)

Substitute Vf for Vs/2 and Γ for (ZL - Zo)/(ZL + Zo).  The resulting equation becomes:

Vdet = Vf*Γ

And therefore:

Γ = Vdet/Vf

One more note regarding the circuit, above:  because R1 and R2 play no role in the impedance seen looking into the test port from the outside world, they can be any value, not just Zo.  The only rule is that their resistance values be the same so that the "simulated" Vf  at the R1/R2 node equals Vs/2.

And why, you might wonder, do R1 and R2 play no role in the port's impedance?  This is simply the result of the Superposition Principle, which states that, when analyzing how a circuit reacts to a voltage or current source, the effects of all other voltage and current sources connected in the circuit should first be removed.

So, to remove the effect of these other source, the other voltage sources are replaced with shorts, and the other current sources are replaced with opens (i.e. the current sources are simply removed).

So, to analyze the impedance that Vr sees as it enters the test port, first short Vs.  Clearly, the two series resistors R1 and R2 are now shorted to ground, and the impedance looking into the bridge's test port from the outside world consists of a single resistor, R3, shunting the port to ground and thus terminating it with an impedance Zo.

Standard Caveat:

As always, I might have made a mistake in my equations, assumptions, drawings, or interpretations.  If you see anything you believe to be in error or if anything is confusing, please feel free to contact me or comment below.

And so I should add -- this information is distributed in the hope that it will be useful, but WITHOUT ANY WARRANTY; without even the implied warranty of MERCHANTABILITY or FITNESS FOR A PARTICULAR PURPOSE.

Repair Log: Fluke 6060B (RF Output On/Off Switch)

 

I recently discovered my Fluke's 6060B's "RF Output On/Off" switch was not working:

This switch is an elastomeric push-button switch, in which a small conductive disk on the backside of the rubber pushbutton "shorts" two contacts on the switch PCB when the pushbutton is pressed.

Chances are that the PCB switch contacts needed to be cleaned, but to do this the Front Panel Assembly must be removed from the 6060B chassis and then disassembled to access the push-button's circuit-board pads.  Section 4B-4 of the Fluke 6060B manual describes a procedure for removing the Front Panel Assembly, but this procedure is incomplete.

Below are the steps required to remove the Front Panel Assembly from the chassis and then disassemble it so that the pushbutton switch contacts can be cleaned: 

Removing the Front Panel Assembly:

Disassembling the Front Panel Assembly:

If there is physical interference between the edge of the PCB and the metal bracket, as shown above, consider filing a small notch in the PCB to allow it to clear the bracket.

Cleaning the PCB's Switch Contacts:

The switch contacts on the PCB can be cleaned with either Isopropyl Alcohol (I used a 99% solution).  They can also be cleaned with distilled water.  In either case, wet the tip of a Q-tip with your preferred solution and gently wipe away any loose residue on the PCB's switch pads.  Use a clean Q-tip to dry the pads.

I would also recommend a gentle cleaning of the conductive disk that is attached to the rubber push-button (it is this disk that completes the switch contact when the pushbutton is pressed).

To test the resistance of the RF Output On/Off switch when it is depressed, place an ohm-meter across the two PCB vias shown in the image, below, and press the switch.  After cleaning, I measured about 1.5K Ohms when the switch was pressed).  Other switches measured in the range of 500 to 1200 ohms.  Note that this resistance seems to be the resistance across the small conductive "pad" on the back of the rubber elastomeric switch.

Reassembly:

To reassemble, perform steps 1 through 9 in reverse order!

A Tip:  

Keep the screws for each step in separate cups.  This will make reassembly much easier.

Standard Caveat:

As always, I might have made a mistake in my equations, assumptions, drawings, or interpretations.  If you see anything you believe to be in error or if anything is confusing, please feel free to contact me or comment below.

And so I should add -- this information is distributed in the hope that it will be useful, but WITHOUT ANY WARRANTY; without even the implied warranty of MERCHANTABILITY or FITNESS FOR A PARTICULAR PURPOSE.

Repair Log: HP 5216A 7-Digit Counter

 

This blog post chronicles my efforts to repair an older HP 5216A Nixie tube counter.

The HP 5216A is a 7-digit counter specified to count frequencies between 3 Hz and 12.5 MHz.  In addition to frequency measurements, it can also perform period measurements, period-average measurements, ratio measurements, totalizing measurements, and time-interval measurements.

Documentation:

Although an Operating-Service Manual for the HP 5216A can be downloaded from the Keysight website, this version is of very poor quality with the schematics essentially unusable.  I would instead recommend purchasing a manual from Artek Manuals.  The one I purchased (and obtained via electronic download) is of excellent quality and at the time of this writing only $12.50.

Issues with my 5216A:

• The counter, when it counts, only counts in "Check" mode (left-hand front panel rotary switch in the "Check" position), but it doesn't count when a signal is applied to the front-panel input BNC.
• Sometimes the counter doesn't count at all, irrespective of mode, and the gate lamp never illuminates.  And sometimes the power doesn't seem to come on.
• The most-significant (MS) digit does not illuminate reliably -- it will briefly flicker on, but remains off most of the time.

Repair Efforts:

1.  Power Supply Recapping:

The serial number of my 5216A has a three-digit prefix of 748, implying it was manufactured in 1967 (i.e. take the first two digits of a four-digit prefix (in this case "07") and add them to "1960").

Given that this counter was almost 60 years old, I decided to recap all of the electrolytic capacitors on the power supply board, using caps from my collection of miscellaneous electrolytic caps.

My replacement caps were often the wrong form factor, having radial leads rather than axial, but I was able to attach them without too much effort.  And if I did not have the exact capacitance or working-voltage specified in the manual for a capacitor, I would choose one with a higher capacitance and/or higher working-voltage rating.

With these caps replaced, the issues listed above still existed.

2.  Only counts in "Check" mode, but not when a signal is attached to the front panel input BNC.

The counter would only count when the left-hand rotary switch was placed in "Check" mode, letting it count the counter's internal frequency reference (or a divided-down product thereof).

To trouble shoot, I applied an external signal to the counter's front panel BNC input and followed it through the circuitry.  It appeared to not make it through IC11 on the Main Board (A4).  

As shown in the 'red' annotation on the schematic, above, the input signal appears at IC11 pin 4, but not at its output, pin 6.

Yet pin 5 was high (i.e. TTL input-floating level of around 2 V), which should have gated the signal on pin 4 through.  And also pin 3 was 0, so the other AND gate was cut off and should not interfere with the propagation of the signal from pin 4 to pin 6.

So most likely IC11 (HP part number 1820-0072) was bad.

P/N 1820-0072 is equivalent to an SN7450.  I was able to obtain one via eBay.  (Note, if you cannot locate an SN7450, you can use an SN7451 in a pinch.  It should support all counter functions except those requiring that the 'Time Interval' switch on the counter's back panel be set to "TIME INT" in lieu of "FREQ-PER").

Replacing IC11 fixed the problem of the counter not counting when a signal was applied to the counter's input.

3.  Intermittent Problems:

Sometimes the counter would not count at all in either "Check" mode or in Normal mode.  Sometimes it did not seem to even turn-on.

All of these problems were traced to wires that had broken at their solder joints.  I'm theorizing a previous owner  (one or more), when trying to repair the counter (there were several repairs that had been made previously to the main board, for example), overstressed the wiring harnesses while disassembling the unit, and these wires eventually broke from over flexing.

Here's an example:

Repairing the various broken wires (there were three or four) fixed the intermittent problems.

4.  Left-most Nixie digit not working.

The Nixies are driven by decoder-drivers ICs, HP part number 1820-0092.  I verified that the input to IC15, the decoder-driver for the MS (Most-Significant) digit was correct (a four-bit BCD-encoded low-true value), but the correct Nixie digit representing that BCD code was not being illuminated.

It looked to me as though the Nixie tube was soldered to the PCB (important note: IT IS NOT!), and so I decided that trying to replace the 1820-0092 would be a better path to take.

Unfortunately, a replacement 1820-0092 is almost impossible to find, so I designed an equivalent circuit using available parts, in this case an SN74141 decoder-driver (or its equivalent, a Russian K155ID1 IC, available on Amazon and eBay), and a 74LS04 hex-inverter to convert the 4-bit low-true BCD code input to high-true for the 74141 (or its equivalent) input.

This circuit is described in this blog post:  https://k6jca.blogspot.com/2024/07/hp-1820-0092-nixie-decoderdriver.html

I removed the suspect 1820-0092 and replaced it with an adapter board on which I'd built the new replacement circuit.  The Nixie still did not work.  Oh oh -- was the problem with my replacement design, or with the Nixie tube, itself?

(Here is an image of the Main PCB with my 1820-0092 replacement circuit installed).

Poking around a bit more, I discovered that the Nixie tubes are NOT soldered onto the PCB, but instead they are inserted into pin-sockets, and so they are easy to remove.  I swapped the bad tube with a working tube.  The problem followed the bad Nixie tube, and I was able to show that my 1820-0092 replacement circuit worked properly for decoding digits 0-9 and blanking the tube for non-valid input BCD codes.

These Nixie tubes (HP part number 1970-0025) display their digits "upside down" and are very difficult to find.  Luckily, I have an old HP 5221B counter that uses the same Nixie tubes, but it is only a 5 digit counter, not 7, and it is spec'd to 10 MHz instead of the 5216A's higher frequency of 12.5 MHz.

So I decided it would be the sacrificial lamb, giving one of its Nixie tubes to replace the bad Nixie in the 5216A.  (Now I'm trying to find a replacement Nixie for the HP 5221B).

Other Notes:

1.  Creating a 1 MHz Reference Frequency for the 5216A from 10 MHz:

The HP 5216A's External Frequency Reference Input Specification is:

• 1 MHz Sine Wave (note: square-wave should be adequate, too).
• 1 V rms into 1000 Ohms (10V rms maximum).

I have a "house" 10 MHz frequency reference (based on GPS) that I use to maintain the frequency accuracy of various pieces of test gear in my lab.  But I would need to divide this down to 1 MHz for the 5216A counter.

Here's the design I came up with.

Schematic Notes:

• C2 AC-couples the 10 MHz signal at the input BNC connector to the input of the inverter (NC7S14M5X).
• Resistors R1 and R2 set  the inverter's input DC level to midway between VCC and GND.
• Diodes D1 and D2 are clamping diodes to clamp overshoots and undershoots on the input signal, with R3 acting as a current limiter.  (The inverter also has integrated internal clamp diodes, but they are limited to 20 mA).  
• U2 is a CMOS inverter with a Schmitt-Trigger Input (to prevent false counting of the counter U1 from noise on the input signal).  I used an NC7S14M5 device because I had it in my junkbox.  A 74AHC1G14, or equivalent, should work just fine.
• U1 is a dual divide-by-10 counter, each counter consisting of two stages:  first a divide-by-5 stage that produces an asymmetrical 2 MHz intermediary signal, and then a divide-by-2 stage that also "squares up" the asymmetric signal; the result being a 1 MHz square wave.  Only one of the two divide-by-10 counters is used.
• C1 AC couples U1's output to the output BNC connector.
• R4 both limits output current and also provides a one-pole low-pass filter in conjunction with C4, removing ringing on the output signal, and its value is not large enough to significantly affect signal level, given the 5216A's input impedance of 1K ohms for the reference signal.  Note, too, that given the low-frequency of operation and the short lengths of coax that might be used to connect the 1 MHz output to a downstream input, R4's value does not need to equal the characteristic impedance of the coax.

Implementation:

I built the circuit on a small perf board that could be inserted into a three-BNC Pomona Box, as shown below.  Note that the NC7S14M5 inverter is in a SOT23-5 package and thus mounted on a small adapter board of its own to make signal connection easier.

The three-BNC Pomona Box housing the circuit is shown below.  Note that the 5.1 VDC power comes from a BNC on the front panel of the HP 5216A counter (a modification added to the counter's front panel by a previous owner), which connects to the counter's internal 5.1 VDC rail.

The 10 MHz input:

And the 1 MHz output:

Below is the counter using the 1 MHz External Reference.  The gray coax cable provides 5.1 VDC from the (added) BNC on the counter's front panel to the divide-by-10 circuitry attached to the External Frequency Reference Input BNC on the counter's rear panel.

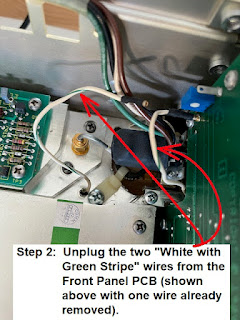
2.  Removing the Main PCB (A4) for repair/troubleshooting.

Unless you have an Operating-Service Manual, it isn't clear how to remove Main PCB to get access to all of the IC pins and components for trouble-shooting or repair.  When mounted within the chassis, significant parts of the PCB are difficult to access with, for example, a scope probe.

But removal of this PCB is actually straightforward.  Per the manual's section 5-14:

First, remove both side panels and the top cover.

Then, remove the transparent colored plastic front-panel window by sliding it out either side of the unit.

Next, reach through the sides of the chassis and gently lift the sides of the main PCB.  Pull the board forward with your fingers.

And after the board is started, remove connector XA4 (the connector on the left-side of the rear of the PCB, when viewed from the front of the counter).

(This works better with two hands -- I was holding my camera with my other hand).

Push or pull the board out of the counter, being careful to keep the board moving in a straight line.

With the PCB now outside of the counter, you can reattach it to connector XA4 for trouble-shooting with power applied.  But there are two important caveats!

First, be sure to place something under the PCB (and behind connector XA4) to prevent either the board or the connector from shorting to the case.

Second, there is high voltage on the board, and it is easy to touch.  (Been there, done that!)  I now wear  gloves when making measurements on the board,

In the image above, several sheets of paper from a yellow legal-pad serve to temporarily isolate the board and connector XA4 from the chassis.

Standard Caveat:

As always, I might have made a mistake in my equations, assumptions, drawings, or interpretations.  If you see anything you believe to be in error or if anything is confusing, please feel free to contact me or comment below.

And so I should add -- this information is distributed in the hope that it will be useful, but WITHOUT ANY WARRANTY; without even the implied warranty of MERCHANTABILITY or FITNESS FOR A PARTICULAR PURPOSE.

HP 1820-0092 (Nixie Decoder/Driver) Replacement

Recently I decided to check out and, if necessary, repair several older HP units that used Nixie tubes for their numeric displays.

The first unit I checked was a 7-digit HP 5216A counter which had a number of problems, including the MS (Most Significant) digit's Nixie tube not illuminating.

While trouble-shooting this problem, I thought that one issue might be with the Nixie Decoder/Driver IC, a custom HP device whose part number is 1820-0092 (shown below in the HP 5216A display PCB).

This device is a 16-pin DIP IC that decodes a 4-bit Binary-Coded-Decimal (BCD) value into one of ten low-true (open-collector) outputs representing the digits 0-9.  Binary values that are outside the range of 0-9 are not decoded and, in these cases, all outputs are left in their undriven state.

Note that the 4-bit BCD values that drive the decoder are low true.  That is, a '0' is represented by an input value of 0b1111 (i.e. 0xF), not 0x0000.  A '1' is 0b1110 (i.e. 0xE), not 0x0001, etc. 

But where do I find a replacement 1820-0092?  This part is no longer manufactured, and if I were to replace it I would need to come up with an equivalent design (unless I could find one on eBay, which I could not).

The TTL SN74141 IC is almost identical in function to the 1820-0092, but its inputs are high-true, not low-true, plus it has a different pin-out.  Unfortunately, this IC is also difficult to find.  However, there is a Russian equivalent IC that can usually be found on eBay or Amazon, the K155ID1, and labelled in Russian as: К155ИД1:

This IC can be used in conjunction with 4 inverters (converting the low-true 4-bit BCD inputs to high-true) to replicate the original function of the 1820-0092, per the schematic, below:

Although the schematic shows a 74141 IC as the decoder/driver, its pin-out is identical to the Russian K155ID1 device that I actually used.

A 74LS04 (hex inverter, from my junk box) is used to invert the four BCD inputs.

Below is my implementation on a small piece of perf-board.  At the far left of this "adapter board," on the under-side of the perf-board, are 16 pins in a DIP pattern that will insert into a socket that I will mount on the HP 5216A's PCB at the location of the defective 1820-0092.  Pin 1 of this 16-pin DIP "plug" is at the upper left.

The K155ID1 is the IC in the middle.  Its pin 1 is at the lower right.  And the SN74LS04 is at the right, with its pin 1 also at the lower right.

Below is the HP 5216A board with the faulty 1820-0092 removed and replaced with a 16-pin DIP socket:

And the following image shows the adapter board mounted in the socket:

Note that the adapter board extends over all or part of two other ICs.  Also, the Nixie tubes are very close to the edge of the adapter board (a reason to keep it small).

That's it!  It seems to work fine.

Standard Caveat:

As always, I might have made a mistake in my equations, assumptions, drawings, or interpretations.  If you see anything you believe to be in error or if anything is confusing, please feel free to contact me or comment below.

And so I should add -- this information is distributed in the hope that it will be useful, but WITHOUT ANY WARRANTY; without even the implied warranty of MERCHANTABILITY or FITNESS FOR A PARTICULAR PURPOSE.

Bringing an ARC-5 Receiver Back to Life, Part 2 (An AC Power Supply)

This post describes an AC power supply I designed and built for powering old military ARC-5 receivers that are either in unmodified condition (i.e. designed for 28VDC operation) or modified for either a 12.6 VAC or 6.3VAC filament voltage)..

Power Supply Requirements:

• +HV (High Voltage):  Must be between 200 and 250 VDC
• LV (Low Voltage):  This is a receiver's filament voltage.  Three different filament voltages (25.2 VAC, 12.6 VAC, and 6.3 VAC) should be available for powering ARC-5 receivers.
• Audio:  RCA jack for attaching an 8-ohm speaker, and an internal Impedance Matching transformer to transform the speaker's 8-ohm impedance to 600 ohms as load for the receiver's audio output signal.
• BFO ON/OFF switch (front-panel mount).
• GAIN Control (front-panel mount).
• All output voltages and control signals available both on a female Octal connector and on an eight-terminal terminal strip.
• Power ON/OFF switch (controls both +HV and LV) and Indicator Lamp (ON when power is ON) both mounted on the front panel.

Schematic:

The schematic is shown below:

Schematic Notes:

• The Transformer's secondary specification is 300 Vrms, center-tapped (Vprimary = 115 VAC, Secondary Load = 86 mA).  Assuming full-wave rectification (i.e. center-tap tied to ground) and a capacitor-only filter (which acts as a peak-detector) the peak DC voltage (and ideally the output voltage, if the capacitor is large enough) should be the peak of one-half of the secondary's Vrms spec, which calculates to be 212 VDC.
• If the secondary's peak voltage is 212 VDC, the diode Peak Reverse Voltage (PRV, or PIV) should be at least 2x 212 VDC, or 424 VDC, plus a good margin to account for AC Line voltage variation, etc.  1N4005 diodes (PRV = 600V) should suffice, but I went with higher-rated diodes (1N4006, PRV = 800V) from my junk box.
• The higher the capacitance attached to the full-wave rectifier, the lower will be the AC ripple on the DC line.  My BC-454-B receiver's frequency varies if +HV changes (the frequency change is *very* roughly 60 Hz per 10V change in +HV), so if +HV had significant ripple, there could be FM'ing of the audio signal.
• The 68K bleeder resistor has a time-constant of 32 seconds in conjunction of 470 uF (and especially important, it was in my junkbox).  And its power dissipation across 212 VDC is about 0.7 watts, so the resistor's 2 watt rating provides a nice margin.
• The Audio transformer's primary will have an impedance of 1.2K ohms if terminated with an 8-ohm load.  The receiver requires a load of 600 ohms, which is available at the primary's center-tap (i.e. its impedance will be one-half of the total primary impedance of 1200 ohms).  Note that the selection of this transformer (a Xicon 42TM003-RC transformer) is discussed further in my BC-454-B post.
• I chose a lamp power-on indicator (with a #44 bulb), instead of an LED to match the era of the ARC-5 receivers.

Front View:

On the left is the POWER ON/OFF switch and above it a panel-lamp (with a #44 bulb) to indicate when power is ON.

The middle switch is BFO ON/OFF.

And the right control is the GAIN potentiometer.

Back View:

Across the top of the back panel is a Terminal Strip consisting of 8 screw terminals.

Below it, from left to right, are:

• Octal socket (with same signals assigned to the same pin numbers as those on the terminal strip)
• Fuse Holder
• RCA jack (connect to an 8-ohm speaker)
• AC Power Connector.

Bottom View, Looking Towards Rear Panel.

(For reference)

At the upper right is the audio impedance-matching transformer (mounted on a piece of perf-board).

Just below it is the 470 uF capacitor, the 68K bleeder resistor, and the two diodes.

Bottom View, Looking Towards Front Panel:

(For reference)

Terminal Strip, Identification of Terminals:

Note the color coding under each screw-terminal's name -- I used the color-code to identify terminals for cabling purposes -- the associated spade terminals (that connect at the power supply side of the cable) are color coded to match their screw terminals.  

For example, the first terminal on the left (Terminal One) is the +HV terminal, and its associated color is BROWN (this might not be obvious from the photograph, but there is a brown line drawn under the "+HV" text.  The associated +HV wire (in the cable bundle) from the receiver that attaches to this screw terminal has its spade-terminal color coded with a matching BROWN.

The next terminal to the right is Terminal Two, and its color is RED.  It is the GND terminal.

The Terminal Numbers increase as we move to the right and the color code increases to match the numbers.  At the far right is the eighth terminal (BFO On/Off).  Per the color code, this should be grey, but I did not have a grey Sharpie, which would have been the appropriate color per the color-code, so I instead colored it with alternating black and white lines.

Note that the numbers assigned to the terminal strip match the pin number of the octal socket.  For example, Terminal One (+HV) of the Terminal Strip connects to the octal socket's pin 1, Terminal Two (GND) to the octal socket's pin 2, etc.

Interconnect Cable (Power Supply Terminal Strip to Receiver J3):

Both ends of the cables that interconnect the Power Supply's Terminal Strip to the Receiver's J3 connector (on the back panel) are color coded.  For example, in the image below, the spade terminal that attaches to the Terminal Strip has a red band, identifying that it should attach to the second terminal (from the left) of the Power Supply's terminal strip (i.e. the GND terminal).

On the other end of the wire is a mini Banana Plug (2.5 mm diameter), which will insert into Pin 1 of the Receiver's rear panel connector, J3.  Note that it has a brown band, identifying that it should be inserted into pin 1 of J3 (i.e. the receiver's GND pin).

I sourced these miniature Banana Plugs, from Amazon:

Note:  not all 2.5mm Banana Plugs are compatible with the receiver's jack.  I purchased a different set (that were only metal -- there was no plastic covers) and they did not fit.  So you might need to do some experimentation.

Interconnect Cable (Power Supply Octal Socket to Receiver J3):

Below is an interconnect cable that uses the power supply's Octal socket in lieu of its terminal strip.  This specific cable is for radios that require a filament voltage of 12.6 V.

Banana Plugs Inserted into a Receiver's J3 connector:

The image below shows six miniature Banana Plugs inserted into a receiver's J3, which is a seven-pin jack.  Note that only J3 pin 5 (+screen grid voltage) is not used, and therefore it does not have a Banana Plug inserted into it.

Receiver J3 Pinout:

The partial schematic, below, identifies the signals at each pin of an ARC-5 receiver's J3 rear panel connector:

Note that pin 5 is not used -- therefore only six miniature Banana Plugs are required.

Measurements:

Given a measured AC line voltage of 118 VAC, I measured the following power supply output voltages:

Connected to a BC-454-B, powered ON, with filaments wired for 28V:

• +HV:  measured (loaded) 212 VDC with 0.15 VAC ripple.
• 25.2 VAC:  measured (loaded) 28.2 VAC.
• 12.6 VAC:  measured (unloaded) 14.1 VAC (13.6 VAC when loaded with an R-27 Receiver).
• 6.3 VAC:  measured (loaded with panel lamp, but no receiver):  7.2 VAC.

Connected to an R-27 receiver, powered ON, whose filaments have been rewired for 12V:

• +HV:  measured (loaded) 212 VDC with 0.15 VAC ripple.
• 12.6 VAC:  measured (loaded) 13.6 VAC.

Notes on the measurements:

• The loaded 25.2 VAC filament voltage (when attached to the BC-454-B) measures 28.2 VAC -- almost 10 percent higher than the recommended filament voltage of 25.2 VAC (for two equal filaments, wired in series).  However, this RMS voltage is essentially the same as the DC voltage used to power the receiver when a Dynamotor is used (28 VDC).  Note, too, that it is close to RCA's recommended voltage tolerance of +/- 10%.  If the AC line voltage were to increase above 118 volts, this 10% specification would be exceeded.

• The loaded 12.6 VAC filament voltage (when attached to the R-27 receiver) measures 13.6 VAC, or 8% above the recommended filament voltage.  If line voltage were to increase, then RCA's 10% maximum variation spec could be exceeded.  

• If it is important to a user that the filament voltages, when loaded, measure to be 25.2, 12.6, and 6.3 VAC when the AC input line voltage is at its nominal value (typically 120 VAC), resistors could be added, within the power-supply, in series with each filament line to provide the necessary voltage drop.  The resistors would probably only be a few ohms each, and probably spec'd at a watt, or less.

Other Notes:

• L14 is in series with the LV (filament) voltage -- does it have an effect on filament voltage?  This part is an RF choke whose inductance is 112 uH.  At 120 Hz its reactance is only 0.084 ohms -- in other words, negligible.

• I strongly recommend covering pin 2 (LV) and pin 3 (+HV) on the Receiver's J2 connector (the Dynamotor connector) to prevent accidentally shorting these pins to ground (or shocking oneself).  Perhaps use heat-shrink tubing?

Standard Caveat:

As always, I might have made a mistake in my equations, assumptions, drawings, or interpretations.  If you see anything you believe to be in error or if anything is confusing, please feel free to contact me or comment below.

And so I should add -- this information is distributed in the hope that it will be useful, but WITHOUT ANY WARRANTY; without even the implied warranty of MERCHANTABILITY or FITNESS FOR A PARTICULAR PURPOSE.