One way to verify the impedance of a common-mode choke (i.e. CM choke) is with a Vector Network Analyzer (VNA), such as the Agilent 8753 series or the popular nanoVNA.
Using such a VNA, a common mistake is to try to determine a CM choke's impedance using an S11 measurement -- this method of determining CM choke impedance can lead to very inaccurate results unless the user is aware of the potential pitfalls and how to correct for them. (Determining CM choke impedance with an S21 measurement is usually a better method.)
An example of an erroneous S11 CM choke measurement is shown in the figure, below. The choke consists of 12 turns around a Mix 33 FT-240 core. (Note that this isn't actually a common-mode choke -- it is simply a choke, but its impedance would be the same as a common-mode choke wound the same way.)
The plot shows a maximum impedance of about 5600 ohms at 7 MHz, after which impedance falls off with frequency.
But does this plot really represent the impedance of the choke?
If I use the G3TXQ S21 method of measuring a common-mode choke (this method is described here and here), the |Z| plot looks completely different, with a peak of 7400 ohms at 25 MHz:
Why the difference? And can I modify my S11 measurement to give the same (or close to the same) results as the S21 measurement?
The Effect of External Capacitance:
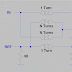
A choke (common-mode or other type) is a two-terminal inductor that, when including its own parasitic capacitance and series resistance, can be modeled as shown in the figure, below:
We can remove the effect of the external parasitic capacitance from an S11 impedance measurement if we know (or can estimate) its capacitance value.
Per the figure, above, the impedance that is actually measured via S11 is:
Z(S11) = Z(DUT) || Z(Cexternal) = 1/(1/Z(DUT) + 1/Z(Cexternal))
We can express this measurement in terms of admittances:
Y(S11) = Y(DUT) + Y(Cexternal),
where:
Y(DUT) = 1/Z(DUT) and Y(Cexternal) = jωCexternal
Rearranging the admittance equations:
Y(DUT) = Y(S11) - Y(Cexternal)
and Z(DUT) is easily found by simply inverting Y(DUT): Z(DUT) = 1/Y(DUT)
If I make the assumption that the external capacitance is around 2 pF (I'm going to use 1.95 pF for the plot, below) and calculate Z(DUT) per the following procedure, I get a "compensated" value of S11 impedance is now much closer to the S21 impedance measurement, as shown by the third line in the plot, below:
Why 1.95 pf? I'll explain this in the next section, below.
R and X versus |Z|:
I'm really not interested in the magnitude of the impedance of a common-mode choke. Although a high magnitude of impedance might seem great, it really tells me nothing about how the choke will behave when installed in an antenna system, because the choke's reactance (whose value is not known if we only examine the magnitude of the impedance) might actually worsen common-mode rejection. (More on a CM choke worsening CM rejection here and here).
Unless you can characterize the impedance of common-mode paths of your antenna system, then measurement of the magnitude of Z (i.e. |Z|) is not very useful.
Instead, calculate the common-mode choke's resistance, as it is this component of the common-mode choke's impedance that is guaranteed to reduce common-mode currents, even if the choke's reactance happens to unfortunately series-resonate with, say, a coax-cable's common-mode path impedance.
For this reason, I always characterize a common-mode choke's impedance in
terms of R and X, and I ignore |Z|.
The plot below shows the DUT's impedance converted into R and X for S11, S21, and the compensated S11 measurements:
Let's examine the plots, above, and get back to the question of why did I chose 1.95 pF for my shunt capacitance value...
Sensitivity of Z, R, and X to External Parasitic Capacitance:
How sensitive is the plot of the "compensated" S11 impedance measurement to the value of the external parasitic capacitance?
In addition to the original S11 and S21 |Z| plots, the figure below has three |Z| plots of the compensated S11 measurement using three different values of capacitance. I've selected 2.0 pF as the "nominal" capacitance, with the other two capacitances being the this nominal capacitance varied by +/- 5% (i.e. 1.9 pF and 2.1 pF). You can see that there is an appreciable change in |Z| at the higher frequencies.
The figure below shows the R and X components for the original S21 plot as well as for the compensated S11 measurement using the two +/- 5% values of external capacitance (i.e. 1.9 and 2.1 pf).
Note that in this example, a change in capacitance of 10 % results in roughly a 20% change in the frequency of peak choke resistance!
Measuring Shunt Capacitance:
I can get an idea of the value of the parasitic shunt capacitance of my S21 Fixture by using the "Y21 method" to calculate three impedances of the fixture: the series impedance between ports 1 and 2, and the shunt impedances (to ground) at either port.
To perform the S-parameter measurements for the Y21 calculations, I first perform a full 2-port VNA calibration in which the THRU standard is a short BNC barrel:
And then I replace this barrel with the "S21 Fixture" and measure the fixture's S-parameters:
The fixture's S-parameters, with shorting wire attached, are:
The figure below shows the fixture's series-impedance (displayed as R and X) as calculated using two methods: G3TXQ's S21 method and the Y21 method.
G3TXQ's method does not take into account the VNA port shunt impedances, which can result in errors, such as the resistance component of the series-impedance going negative, as shown in the next plot. But if I use the Y21 method, I can model the measurement circuit as a three-element Pi network and calculate both the series impedance and the two shunt impedances:
Using the Y21 method we see that the fixture, when shorted with a wire, looks like a series impedance of 0.21 + jω200e-9 ohms (i.e. it is about 200 nH of inductance in series with about 0.2 ohms of resistance):
And the shunt capacitances at either port are about 2.1 pF:
Note that these shunt capacitances are modeled as lumped-elements, approximating what is actually happening with the fixture's distributed circuit.
Impedance Measurement using the Y21 Method:
Let's use the Y21 method and calculate the series and shunt impedances when my 12-turn choke is connected to the S21 fixture.
The figure below shows R and X for the choke as calculated from S11, S21, S11 compensated for external port capacitance (1.95 pF), and the Y21 method.
The figure below shows the external shunt capacitance values calculated with the Y21 method. Note that these are now closer to 3 pF, rather than 2 pF. This is possibly due to distributed coupling from the physical structure of the choke to ground, which then becomes part of the 3-component lumped-element Y21 circuit approximation.
Conclusions:
1. Stray shunt capacitance can greatly affect the measured impedance of a common-mode choke if measuring it using a VNA's S11 measurement. If you know the approximate value of your stray shunt capacitance, you can remove its effect from the measurement by converting impedances to admittances, and then subtracting from the measured admittance the admittance of the stray shunt capacitance.
But if you do not have a reasonable idea of what your stray capacitance value is, your results might not represent the choke's actual impedance.
And this is exactly the problem with the S11 method of measuring common-mode choke impedances -- what is the shunt capacitance?
2. G3TXQ's S21 measurement method provides a better measure of common-mode choke impedance than S11 measurements. But the Y21 method of measuring impedance (from an S21 measurement) is superior to G3TXQ's method.
A 3-Port Method for Characterizing Baluns: http://k6jca.blogspot.com/2020/06/another-method-to-characterize-baluns.html
I might have made a mistake in my designs, equations, schematics,
models, etc. If anything looks confusing or wrong to you, please feel
free to comment below or send me an email.
Also, I will note:
This
design and any associated information is distributed in the hope that it
will be useful, but WITHOUT ANY WARRANTY; without even the implied warranty
of MERCHANTABILITY or FITNESS FOR A PARTICULAR PURPOSE.