During a recent conversation with a fellow ham, my interest was piqued when
he mentioned the physical construction of the Directional Couplers contained
in the slugs used by Bird Wattmeters.
At the time, I didn't know their principle of operation and I wondered if they were similar to other types of Directional Couplers I'd investigated, such as the Bruene coupler or Tandem Match coupler. That is, at any point along a transmission line, there is a voltage V across the transmission line and current I through that point, and these directional couplers use a "sample" of both this voltage and current to determine forward and reflected power.
And most importantly, given the small dimensions of the Bird slugs, I wondered if their operation could be analyzed with straight-forward lumped-element circuit analysis.
So I started poking around the web, looking for further information as to how the Bird slugs operate. Here's one explanation that I found:
"The Bird directional coupler is a sample loop with a matched resistance at one end and a detector at the other end. Current flowing in one direction heats the resistance. Current flowing in the other direction deflects the meter."Another explanation was a variation on the previous one, but with an induced traveling wave moving down a parallel transmission line (formed by the slug's pickup loop) either towards the meter or towards the resistor terminating this secondary, parallel transmission line.
I love the simplicity of these two explanations and they make intuitive sense. But are they correct?
Note that they both state that the undesired wave (or current) flows towards the resistor at the end of the pickup loop where it is dissipated. And the desired wave (or current) flows in the opposite direction, towards the meter and deflecting it.
Therefore, if we want to measure the forward wave, the induced forward wave needs to travel towards the meter end of the loop.
It is critical to note that this induced wave must be moving in the same direction as the main wave. So, if measuring forward power, the meter should be at the end of the sensing loop closest to the load, and the terminating resistor should be at the other end of the loop, closest to the source.
[If the induced forward wave were to travel in the opposite direction of the main wave, then there's a huge issue with causality. Imagine this example: the main transmission line is very very long (let's pick a length, say, several light-years), and we put next to this line a second transmission line of the same length that is going to be our "pickup sensor" line. If the induced wave really traveled in the opposite direction, then, if we start a wave traveling down our main line from left to right, the induced wave would have to start simultaneously at the far end of the second "pickup" transmission line if it's going to be moving in the opposite direction, from right to left.]
So, to recap a requirement that must be satisfied if the "traveling wave" explanation were true, the meter end of the loop must be towards the load and the resistor end towards the source when measuring forward power.
But here's the problem. In actuality, when measuring forward power, the meter end of the loop is towards the source and the resistor end is towards the load. The termination resistor is actually at the opposite end of the loop from where it should be if the "induced traveling-wave" explanation were correct!
And therefore, the "induced traveling-wave" explanation cannot be correct!
(You can verify the position of the resistor by taking a look at the resistor locations in a "Monimatch" type of coupler (which is similar to a Bird sensor but with larger dimensions and cost reduced). You will see the termination resistors placed as I described -- towards the antenna for forward power and vice-versa for reflected power.)
So -- is there a better way to analyze the operation of a Bird slug?
Before I get to the meat of this blog post, I'll add my own philosophy, which is this: if the dimensions of a circuit are very small compared to the wavelength of the frequencies over which it is to operate, then it can (and should) be analyzed using straight-forward lumped-element circuit analysis.
And I'll note that the dimensions of a bird slug are very much less than the wavelengths it's operating at, especially at HF.
I'll add this important note:
The Bird wattmeter, whose sensor can be considered a "lumped-element" device, is only looking at the voltage and current present at its output port. It has no idea what the load is, or even how the load is connected to the port. The load might be a resistor or other component simply clipped onto the output connector with test leads, or it might be a length of transmission line with a load (either known or unknown) at its other end.
Irrespective of what the load is (transmission line, clipped-on component, or whatever), the Bird Wattmeter gives us Power readings for what we assume is a Forward wave or, if the sensor is rotated, for the Reflected wave. It is important to remember: these Power readings should only be interpreted as representing actual Forward and Reflected Power when the Wattmeter is connected in a transmission line with the same characteristic impedance, Zo, as the Wattmeter's designed-for target impedance!
Therefore, for this discussion I will assume that the Bird Wattmeter is inserted into a transmission line of the designed-for (target) characteristic impedance.
Analysis of the Bird Slug's Directional Coupler:
In understanding how the Bird circuit works, there's no better place to start than with the Bird patent itself:
Patent US2852741
Directional Wattmeter, J. R. Bird et al. Granted 16 September
1958
I'll start this discussion by noting that the patent is very readable, and I would recommend anyone interested in the subject to click on the link above to download it.
And let's also note that, regarding the analysis of the slug's operation, the patent states:
"Dimensions are kept to a minimum, much less than the wave length of the energy transmitted, and in considering the theory of operation it is satisfactory to refer to lumped impedances rather than evaluating the distributed parameters." [Emphasis mine, k6jca](I'll add that the patent then continues, "In practice, however, it has been necessary to supplement theoretical calculations with empirical methods of testing in making corrections for distributed capacitance and distributed inductance.") ["Strays" is my "official engineering term" for the latter.]
But lumped-element analysis is how Bird presents their operation, and we shall follow that line. Note that I will use the concepts of Forward and Reflected waves for this analysis, but later in this post I will present an alternative analysis that does not use waves at all.
First, to get an idea of how a Bird slug is constructed, here are some illustrations from the patent.
(click on image to enlarge)
And, again from the patent, here's an equivalent-circuit schematic (Fig. 13) of the components.
(click on image to enlarge)
For my analysis, let me simplify the patent's Figure 13 so that it looks like this:
(click on image to enlarge)
Before we start, some assumptions and definitions:
- V is the voltage across the transmission line at the point of measurement, and I is the current along the transmission line (e.g. on the coax-cable center conductor) at the point of measurement.
- "jw" in the equations below represents j*omega, where omega = 2*pi*Frequency (just in case it isn't obvious).
- M is the Mutual Inductance between the coax center-conductor (carrying the RF current) and the pickup's loop-wire. It can be represented by an induced voltage source in the loop wire of value jw*M*I.
- C is the capacitive coupling between the coax center-conductor and the pickup loop wire. It's actually a distributed capacitance, but this distribution can be "biased" towards the resistor end of the loop with extra copper at that end (item 150 in Fig. 13).
- The induced-voltage source in the pickup wire creates a loop-current from the "+" terminal of the voltage source through C1 to ground, then up from ground through R, and then back along the loop wire to the "-" terminal of the voltage source. For the equations in the patent to hold true, it is important that this loop current *not* create a significant voltage drop through R, compared to R's function as a voltage-divider in combination with C. So we will assume C1 is very small Therefore C1 can be ignored because its impedance is very high. (In reality this isn't the case, but we need to assume this to replicate the patent equations which do not contain any C1 terms).
- It's assumed that the self-inductance of the loop wire is negligible, that is, its impedance is so low that the voltage drop across it can be ignored.
- I'm ignoring the effect of the capacitive sleeve around the resistor R (see text further down).
Assuming that the loop-current created by the induced-voltage source creates a negligible voltage drop across R, then the voltage at Node A (in the drawing above) is:
V(node A) = V*R / ((1/jwC)+R)
because it's a voltage divider.
If we assume that the impedance of the capacitor C is much larger than R (because it is a very small capacitance), then the equation can be simplified to:
V(node A) = V*jw*C*R
The wattmeter measures voltage at Node B. Using the assumptions listed above, the voltage at Node B is simply the sum of the voltage at Node A plus the value of the induced-voltage source:
V(node B) = V*jw*C*R + jw*M*I
Rearranging:
V(node B) = jw*(V*R*C + M*I) (equation A)
Which is exactly the same as Equation 2 in the patent (except I use of "V" in lieu of "E"). So my analysis tracks theirs.
Note that if the sensor (i.e. slug) is rotated 180 degrees so that R is now closest to the source side of the line, rather than the load side:
(click on image to enlarge)
Equation A now becomes:
V(node B) = jw*(V*R*C - M*I) (equation B)
Note the minus sign! This is because, although the voltage at Node A
does not change polarity, the polarity of the induced-voltage source is now
flipped with respect to Node A: the "+" terminal of the induced
voltage source is still to the left in the drawing because current on the
coax center-conductor is still flowing from left-to right. And if we
now sum up these voltages to determine the voltage at Node B, we find that
they subtract, rather than add.
Continuing on...
Note that, at any point along a transmission line (such as our measurement
point), I = V/Zo, where V is the voltage across the line at that point and
Zo is the characteristic impedance of the transmission line. If we
substitute this equality into equations A and B, we get:
V(node B) = V*jw*(R*C + M/Zo) (equation C)
V(node B) = V*jw*(R*C - M/Zo) (equation D)
If you take a look at the patent, you'll notice that the component values are selected to meet the patent's Equation 4, which is:
R*C = M/Zo = K
Where K is a designer-defined constant.
What happens if we substitute this equality for K into equations C and D? Equation C (sensor in original position) becomes:
V(node B) = 2*V*jw*K (equation E)
and Equation D (sensor rotated 180 degrees) becomes:
V(node B) = 0 (equation F)
In other words, if the wave is only moving from Left to Right and there
is no wave moving from Right to Left (i.e. there's only a forward wave and
no reflections from the load), then we get equations E and F above.
What happens if we now switch positions of load and source on the line in my drawing above (source now to right side, load to left side), then equations E and F would become:
V(node B) = 0 (equation E')
V(node B) = 2*V*jw*K (equation F')
Now, with a wave only moving from Right to Left (no reflections from the
left-side load), we now read 0 volts when before we read 2*V*jw*K, and,
when the sensor is rotated 180 degrees, it now reads 2*V*jw*K, when
previously it had read 0 volts.
Continuing on...
We can express the equation for Node B (normal and rotated positions) in terms of Vfoward (Vfwd) and Vreverse (Vref) -- waves simultaneously traveling on both directions on the line. E.g. source on left of drawing and unmatched load on right.
First let's bring back equations A and B (sensor in "normal" position, then sensor rotated 180 degrees):
V(node B) = jw*(V*R*C + M*I) (equation A)
V(node B) = jw*(V*R*C - M*I) (equation B)
V(node B) = jw*(V*R*C - M*I) (equation B)
V = Vfwd + Vref (equation G)
I = Ifwd - Iref
Note, too, that on the transmission line Ifwd and Iref are defined as follows:
Ifwd = Vfwd/Zo
Iref = Vref/Zo
Therefore:
I = Vfwd/Zo - Vref/Zo (equation H)
Substituting Equations G and H into A and B and keeping in mind that R*C =
M/Z0 = K, we get the following very important equations:
When the sensor is rotated so that its resistor R is towards the load, we
only measure Vfwd:
V(node B) = Vfwd * 2 * K * jw
And when the sensor is rotated so that its resistor R is towards the
source, we only measure Vref:
V(node B) = Vref * 2 * K * jw
Also, because we know that power is related to the square of the voltage, if
we know the voltage, we can easily calculate both forward and reflected
powers. Voila, we have a directional wattmeter!
Note that these equations for V(node B) contain the term "jw" (which is j*omega, where omega = 2*pi*F). The omega term (2*pi*F) means that this voltage increases at 6 dB per octave and thus the sensor is not flat across frequency. Bird's compensation techniques to flatten the response are discussed in the patent (see the text I've included below), but they are not part of Bird's analytical equations presented in the patent.
Analyzing the Bird Wattmeter in a "Non-Transmission Line" Environment:
We can actually analyze the operation of the Bird element without resorting to Forward and Reverse waves. After all, the above analysis was done using lumped elements, and because of this we can also analyze operation in terms of simple voltages and currents in lieu of waves.
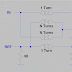
So instead of using forward and reflected waves, as we did above, let's just define V to be the source voltage, which is also the voltage across the load connected to the wattmeter's "output" connector. And "I" is the current being delivered to the load.
The load itself will be an unknown impedance, let's call it "Zload". And we'll attach it to the output connector of the wattmeter with a couple of short clip leads haphazardly draped on the workbench -- no coax.
(click on image to enlarge)
So, from the image above we know that:
I = V / Zload
Recall equations A and B. They still apply for this analysis. For the sensor oriented with R towards the load:
V(node B) = jw*(V*R2*C + M*I) (equation A)
And for the sensor rotated 180 degrees (R towards source):
From our previous analysis, we know that R1, R2, C and M were all chosen
for operation with a 50 ohms system. That is:
K = R1*C = R2*C = M/Zo = M/50
Which is to say that the design parameters were such that:
M = 50 * K
So now let's substitute into equations A and B our equations for "I" and "M". The result, for the voltages measured at the "FWD" and "REF" ports, is:
So now let's substitute into equations A and B our equations for "I" and "M". The result, for the voltages measured at the "FWD" and "REF" ports, is:
Sensor with R towards load:
V(node B) = V*jw*K*(1 + 50/Zload)
Sensor rotated with R towards source:
V(node B) = V*jw*K*(1 - 50/Zload)
As a quick check, what happens if Zload = 50 ohms? We get:
V(node B, "forward" orientation) = V*jw*2*K
V(node B, rotated 180 degrees) = 0
Exactly what we expect.
One interesting conclusion from these equations: The Bird Wattmeter will not accurately indicate forward power if the load is not 50 ohms.
Here's an example:
Suppose the meter is calibrated so that, when V(node B) = 2 volts, this represents 0.02 watts into 50 ohms.
In other words, for this example I'm defining the quantity "jw*K" to be equal to 1, and therefore a voltage "V" that is 1 volt, across a load of 50 ohms, generates a voltage of 2 volts at Node B. That is, for this example our equation for the sensor in its normal (forward power) rotation is:
V(node B) = V*(1 + 50/Zload)
Now, let's terminate the meter with 150 ohms and adjust our source voltage so that V(node B) again reads 2 volts. The meter will again show 0.02 watts. But is this the power being dissipated by the load?
Let's first calculate the voltage "V" across the load. From the equation above, we get:
2 = V*(1+50/150)
Therefore V = 1.5 volts
The actual power being dissipated across the load is calculated as P(load) = V2/Zload. Given V = 1.5 and Zload = 150 ohms, resistive, then:
P(load) = 0.015 watts
So, our meter tells us that the "Forward" Power is 0.02 watts, but in fact the actual dissipation across the load (now 150 ohms) is 0.015 watts.
Interestingly, if the sensor is then rotated 180 degrees, we apply the equation
V(node B) = V*(1 - 50/Zload)
We know that "V" is 1.5 volts and Zload is 150 ohms, so V(node B) with the sensor in the "Reflected" Power orientation should now be 1 volt.
What power would 1 volt correspond to? Well, it is one-half of 2 volts, which means that the power when V = 2 volts has been decreased by 6 dB. So if 2 volts is calibrated to be 0.02 watts, then 1 volt would be a quarter of that, or 0.005 watts.
From these two measured power values, we can see that the actual power dissipated across our 150 ohm load is the measured P(forward) minus the measured P(reverse), which is 0.015 watts.
But there really isn't any power being reflected back. Remember, at the start of this non-Transmission Line analysis I stated that the resistive load was connected to the meter with a couple of short wires routed willy-nilly from meter to load. I'll quote G3YNH:
"[The bridge] can only infer the existence of reflected power from the difference between the actual load impedance and the target load impedance. To understand this point, consider an SWR bridge designed to balance when the load is 50+j0Ω. If we connect this bridge directly [emphasis mine, k6jca] to a 100Ω load resistor, it will declare an SWR of 2:1. The resistor is not reactive however, and so will absorb all of the power delivered to it and reflect none. The 2:1 SWR reading is only true when the bridge sees an impedance magnitude of 100Ω (or 25Ω) at the input to a 50Ω transmission line. The bridge is just an impedance bridge, it has no special psychic powers, and its readings are only true when it is inserted into a line having the same characteristic resistance."And with that, I'll end the post with the following notes...
Other notes on the circuit, from the patent:
Regarding the unlabeled inductor at the left end of the pickup wire in Figure 13, I didn't find any mention of it in the patent text (but I easily could have missed it). I suspect this represents the self-inductance of the wire and that it is so small that, for this analysis, it is unimportant (and thus unlabeled). But maybe it represents the Mutual inductance. I don't know.
Patent text regarding capacitor C1:
"One of the features which characterizes the present invention is the supplementing of the sampling circuit of the loop and resistor of known instruments by a frequency compensating capacitor used in association with the diode contact rectifier and series connected in the resistor-loop circuit. This supplemental capacitor is connected in parallel relation to the diode circuit which includes the galvanometer or other indicator external to the high frequency components and is of much greater capacitance that the capacitance C coupling the loop and other components of the sampling circuit to the transmission line. The effect of such compensating capacitor is to give broad frequency band operation to directional couplers and the like of the type referred to. It is believe that, since the generated voltage in the loop resistor circuit is proportional to frequency (Eq. 2) and the impedance of the supplemental capacitor is inversely proportional to frequency, the output voltage across the capacitor can be made to remain constant, or substantially so, by suitable selection of values and provided the total impedance of the circuit remains constant."
Capacitor C1 "tunes or resonates the loop circuit in the frequency band and at the sensitivity for which the particular pickup unit is designed. The loop circuit, thus heavily loaded by the capacitor C1, exhibits broadband characteristics by reason of the resistance R which flattens the response curve of the circuit."And...
"This capacitive loading of the resistive loop circuit by the series capacitor C1 is one of the distinguishing characteristics of the present invention and provides wide frequency band operation."
Patent text regarding capacitor C4:
Capacitor C4 [also called C-4 in the patent -- k6jca] is a metal sleeve around resistor R (and insulated from it). C-4 "functions to modify the loop-resistor circuit and obtain several beneficial results, chiefly improved directivity characteristics, but also so-called "flat" response over a wide frequency band."And...
"Placing the modifying capacitance C-4 across the resistor R of the loop-resistor sensing combination in the manner described distributes the capacitance along the length of the resistor and has the effect of improving the balance and directivity of the instrument. With this arrangement a directivity of over 40 decibels is obtained in the instrument described over a wide frequency range having a ratio of at least 2 1/2 to 1 and having satisfactory directivity over even wider frequency ranges, as wide as 5 to ratio being possible, whereas the same instrument without the modifying capacitor has less than about 25-35 decibels of directivity over the same range of frequencies."
Patent text regarding the selection of R and C1 (a.k.a. C-1 in the patent):
"In the selection of the resistor R of the loop-resistor combination and the supplemental or compensating resistor C-1 for these several units empirical methods must be used in conjunction with calculations to satisfy the requirements of Equation 4. The compensating capacitor C-1 is not the "C" of this equation ["C" is the capacitive coupling between line and loop -- k6jca] but must be chosen in relation to the components concerned in Equation 4 to obtain the desired "flat" response of the meter over a wide frequency band."
And patent text regarding the loop and both "M" and "C," the mutual inductive coupling and capacitive coupling between line and loop:
"In balancing Equation 4 the size or diameter of the wire comprising the loop 105 is an influencing factor. Increasing the wire size increases capacitance C between the loop and the inner conductor 10 [see patent figure 13 -- k6jca] of the transmission line, whereas decreasing the wire size decreases such capacitance, the mutual inductance M remaining substantially the same. If desired, a plate 150 [patent figure 8 -- k6jca] of suitable area may be soldered to the straight portion 106 [patent figure 8 -- k6jca] of the loop wire in a plane parallel to the axis of the line to increase the capacitance coupling."
Other related Bird patents:
Patent US2891221, Standing Wave Indicator, J. R. Bird et al. Granted 16 June 1959
Patent 4080566A, RF Directional Wattmeter, Mecklenburg (assigned to Bird Electronic Corporation). Granted 21 March 1978
Links to my Directional Coupler blog posts:
Notes on the Bruene Coupler, Part 2
Notes on the Bruene Coupler, Part 1
Notes on HF Directional Couplers (Tandem Match)
Building an HF Directional Coupler
Notes on the Bird Wattmeter
Notes on the Monimatch
Notes on the Twin-lead "Twin-Lamp" SWR Indicator
Calculating Flux Density in Tandem-Match Transformers
And some related links from my Auto-Tuner and my HF PA posts:
Auto Tuner, Part 5: Directional Coupler Design
Auto Tuner, Part 6: Notes on Match Detection
Auto Tuner, Part 8: The Build, Phase 2 (Integration of Match Detection)
HF PA, Part 5: T/R Switching and Output Directional Coupler
Other references of generally interest:
http://www.g3ynh.info/zdocs/bridges/Xformers/part_1.html great discussion on current-transformers for directional coupler applications
http://www.g3ynh.info/zdocs/bridges/Xformers/part_2.html Part 2 of current-transformers
http://www.g3ynh.info/zdocs/bridges/Xformers/part_3.html And part 3, the last part, of current-transformers
http://www.g3ynh.info/circuits/diode_det/index.html Diode detectors!
http://www.g3ynh.info/zdocs/bridges/index.html Indexes numerous topics. Lots of great info to be found here!
http://www.richtek.com/assets/AppNote/AN008_EN/AN008_EN.jsp Common-Mode choke model
Final Caveats:
As always, I might have made a mistake in my equations, assumptions, or interpretations. If you see anything you believe to be in error, or if anything is confusing, please feel free to contact me.
Notes on the Bird Wattmeter
Notes on the Monimatch
Notes on the Twin-lead "Twin-Lamp" SWR Indicator
Calculating Flux Density in Tandem-Match Transformers
And some related links from my Auto-Tuner and my HF PA posts:
Auto Tuner, Part 5: Directional Coupler Design
Auto Tuner, Part 6: Notes on Match Detection
Auto Tuner, Part 8: The Build, Phase 2 (Integration of Match Detection)
HF PA, Part 5: T/R Switching and Output Directional Coupler
Other references of generally interest:
http://www.g3ynh.info/zdocs/bridges/Xformers/part_1.html great discussion on current-transformers for directional coupler applications
http://www.g3ynh.info/zdocs/bridges/Xformers/part_2.html Part 2 of current-transformers
http://www.g3ynh.info/zdocs/bridges/Xformers/part_3.html And part 3, the last part, of current-transformers
http://www.g3ynh.info/circuits/diode_det/index.html Diode detectors!
http://www.g3ynh.info/zdocs/bridges/index.html Indexes numerous topics. Lots of great info to be found here!
http://www.richtek.com/assets/AppNote/AN008_EN/AN008_EN.jsp Common-Mode choke model
Final Caveats:
As always, I might have made a mistake in my equations, assumptions, or interpretations. If you see anything you believe to be in error, or if anything is confusing, please feel free to contact me.


















2 comments:
I wonder what the Telewave "slugless" meters use.
Guess I'll have to take mine apart and see!
Great job circuit analysis, congratulations and thank you very much for sharing this wonderful information......73'
Post a Comment